An
experimental evidence on the validity of third Smarandache conjecture.
Felice Russo
Micron Technology Italy
Avezzano (Aq) – Italy
Abstract
In this note we report the results regarding the
check of the third Smarandache conjecture on primes [1],[2] for 
and 
. In the range analysed the
conjecture is true. Moreover, according to experimental data obtained,
it seems likely that the conjecture is true for all primes and for all
positive values of k..
Introduction
In [1] and [2] the following function has been defined:
where 
is the nth prime and k is a positive integer. Moreover in the above mentioned
papers the following conjecture has been formulated by F. Smarandache:
for 
This conjecture is the generalization of the Andrica
conjecture (k=2) [3] that has not yet been proven. The Smarandache conjecture
has been tested for 
,
and in this note the result of this search is reported. The computer code
has been written utilizing the Ubasic software package.
Experimental Results
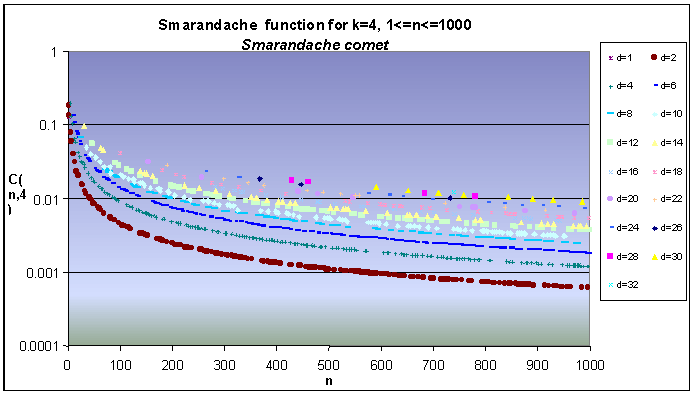
In In the following graph
the Smarandache function for k=4 and n<1000 is reported. As we can see
the value of C(k,n) is modulated by the prime’s gap indicated by 
.
We call this graph the Smarandache "comet".
In the following table, instead, we report:
 | the largest value Max_C(n,k) of Smarandache function for and
 |
| the difference  between
2/k and Max_C(n,k) |
| the value of that maximize
C(n,k) |
| the value of 2/k |
|
k |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
9 |
10 |
|
Max_C(n,k) |
0.67087 |
0.31105 |
0.19458 |
0.13962 |
0.10821 |
0.08857 |
0.07564 |
0.06598 |
0.05850 |
|
|
0.32913 |
0.35562 |
0.30542 |
0.26038 |
0.22512 |
0.19715 |
0.17436 |
0.15624 |
0.14150 |
|
|
7 |
7 |
7 |
7 |
7 |
3 |
3 |
3 |
3 |
|
2/k |
1 |
0.666.. |
0.5 |
0.4 |
0.333.. |
0.2857.. |
0.25 |
0.222.. |
0.20 |
According to previous data the Smarandache conjecture
is verified in the range of k and
analysed due to the fact that 
is always positive.
Moreover since the Smarandache function falls
asymptotically as n increases it is likely that the estimated maximum
is valid also for 
.
We can also analyse the behaviour of difference
versus the k parameter that
in the following graph is showed with white dots. We have estimated an
interpolating function:
for k>2
with a very good 
value
(see the continuous curve). This result reinforces the validity of Smarandache
conjecture since:
New Question
According to previous experimental data can we reformulate the Smarandache conjecture with a more tight limit as showed below?
where
and 
is the Smarandache constant
[4],[1].
References
[1] See http://www.gallup.unm.edu/~smarandache/ConjPrim.txt
[2] Smarandache, Florentin, "Conjectures which Generalize Andrica's Conjecture", Arizona State
University, Hayden Library, Special Collections, Tempe, AZ, USA.
[3] see: "Andrica’s conjecture" in http://www.treasure-troves.com/math/
[4] N. Sloane, Seq. A038458 ("Smarandache Constant" = .5671481302020177146468468755...)
in <An On-Line Version of the Encyclopedia of Integer Sequences>,
http://www.research.att.com/cgi-bin/access.cgi/as/njas/sequences/ eishis.cgi.