SuperHyperStructure & Neutrosophic SuperHyperStructure
 

        The n-th powersets that are the bases o the SuperHyperStructure and Neutrosophic SuperHyperStructure [2, 3, 15], together with their particular cases such as: SuperHyperAlgebra and Neutrosophic SuperHyperAlgebra (endowed with SuperHyperOperations and SuperHyperAxioms) [2016, 2022], SuperHyperGraph (including SuperHyperTree) and Neutrosophic SuperHyperGraph (including Neutrosophic SuperHyperTree) [2019 - 2022], SuperHyperSoft Set, SuperHyperFunction and Neutrosophic SuperHyperFunction [2022], SuperHyperTopology (all built on the powersets of P(H), or Pⁿ(H), for n ≥ 1) and Neutrosophic SuperHyperTopology [2022] were all founded by Smarandache [2] and developed between 2016 - 2024.

        Definition: A SuperHyperStructure is a structure built on the n-th PowerSet of a Set H, for n ≥ 1, as in our real world {because a set (or system) H (that may be a set of items, an organization, country, etc.) is composed by sub-sets that are parts of P(H), which in their turn are organized in sub-sub-sets that are parts of P(P(H)) = P²(H), then in sub-sub-sub-sets that are parts of P³(H), and so on, Pⁿ⁺¹(H) = P(Pⁿ(H) }.

    The powerset P(H) means all non-empty and empty subsets of H, including the empty set ( ф ), which represents the indeterminacy that occurs into the set H (as in our real world where we deal with unclear/indeterminate information in any space/set); and similarly for Pⁿ(H). 

    While P_*(H) means all non-empty subsets of H, or P_*(H) = P(H) - ф. And similarly for P_*ⁿ(H).

        A structure built on P_*ⁿ(H) is called a SuperHyperStructure (has no indeterminacy), while built on Pⁿ(H) it is called Neutrosophic SuperHyperStructure (does have indeterminacy).

        In a SuperHyperStructure we deal with SuperHyperAxioms, SuperHyperLaws, SuperHyperTheorems, SuperHyperProperties, SuperHyperOperators, etc. Similarly for a Neutrosophic SuperHyperStructure.

        1. "Hyper" and "Super" prefixes

The prefix “Hyper” (Marty [1], 1934) stand for the codomain of the functions and operations to be P(H), or the powerset of the set H.

While the prefix “Super” (Smarandache [2], 2016) stands for using the Pⁿ(H), n ≥ 2, or the n-th PowerSet of the Set H {because the set (or system) H (that may be a set of items, a company, institution, country, region, etc.) is organized in sub-systems that are part of P(H), which in their turn are organized in sub-sub-systems, that are part of P(P(H)) = P²(H) and so on} in the domain and/or codomain of the functions and operations and axioms.

           2. SuperHyperStructure

A SuperHyperStructure [2, 2016] is a structure built on the n-th powerset P_*ⁿ(H) of a non-empty set H, for integer n ≥ 1, whose SuperHyperOperators are defined as follows:

#_SHS :  (P_*^r(H))^m ―> P_*ⁿ(H),

where P_*^r(H) is the r-powerset of H, for integer r ≥ 1, while similarly P_*ⁿ(H) is the n-th powerset of H,

and the SuperHyperAxioms act on it.

Indeterminacy is not allowed on this structure.

            3. Neutrosophic SuperHyperStructure

A Neutrosophic SuperHyperStructure is a structure built on the n-th powerset Pⁿ(H) of a non-empty set H, for integer n ≥ 1, whose Neutrosophic SuperHyperOperators are defined as follows:

#_SHS :  (P^r(H))^m ―> Pⁿ(H),

where P^r(H) is the r-powerset of H, for integer r ≥ 1, while similarly Pⁿ(H) is the n-th powerset of H,

and the SuperHyperAxioms act on it.

Indeterminacy is allowed on this structure.

        4. History: From classical Structures and HyperStructures to SuperHyperStructures

        (i) Classical Structure

A classical Structure is built on a non-empty set H, whose classical Operations #_S are defined as:

  #_S :  H^m ―> H, for integer m ≥ 1,

and with classical Axioms acting on it.

        (ii) Classical HyperStructure

A classical HyperStructure (Marty [1], 1934) is built on a non-empty set H, whose HyperOperations are defined as:

#_HS :  H^m ―> P_*(H), where P_*(H) is the powerset of H, without the empty set.

and the HyperAxioms acting on it.

        (iii) Neutrosophic HyperStructure

The Neutrosophic SuperHyperStructure is an extension of the Classical HyperStructure, because it allows indeterminacy (uncertainty, unknown), denoted by the empty set ( θ ), into the the powerset of H, that it is denoted by P(H), without _*

        (iv) SuperHyperStructure

A SuperHyperStructure is built on n-th powerset Pⁿ(H) of a non-empty set H, for integer n ≥ 1, whose SuperHyperOperators are defined as follows:

#_SHS :  (P^r(H))^m ―> Pⁿ(H),

where P^r(H) is the r-powerset of H, for integer r ≥ 1, while similarly Pⁿ(H) is the n-th powerset of H,

and the SuperHyperAxioms act on it.

Indeterminacy is not allowed in this structure.

        (iv) Neutrosophic SuperHyperStructure

A Neutrosophic SuperHyperStructure is built on n-th powerset Pⁿ(H) of a non-empty set H, for integer n ≥ 1, whose SuperHyperOperators are defined as follows:

#_SHS :  (P^r(H))^m ―> Pⁿ(H),

where P^r(H) is the r-powerset of H, for integer r ≥ 1, while similarly Pⁿ(H) is the n-th powerset of H,

and the SuperHyperAxioms act on it.

Indeterminacy (denoted by the empty set θ ) is allowed in this structure.

      (v) The n-th powerset of a set H with Indeterminacy (denoted by the empty set θ)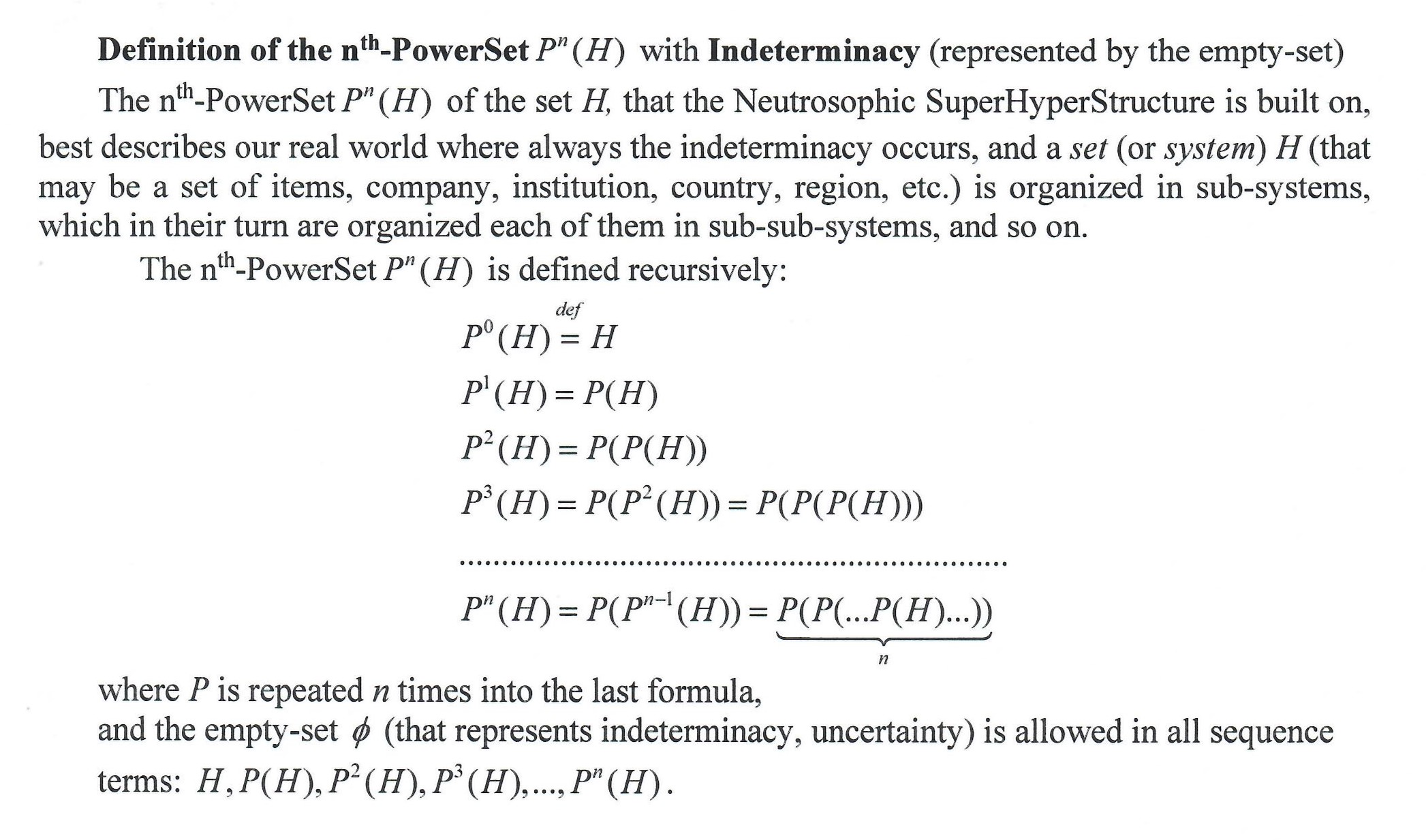

                 (vi) The n-th powerset of a set H without Indeterminacy

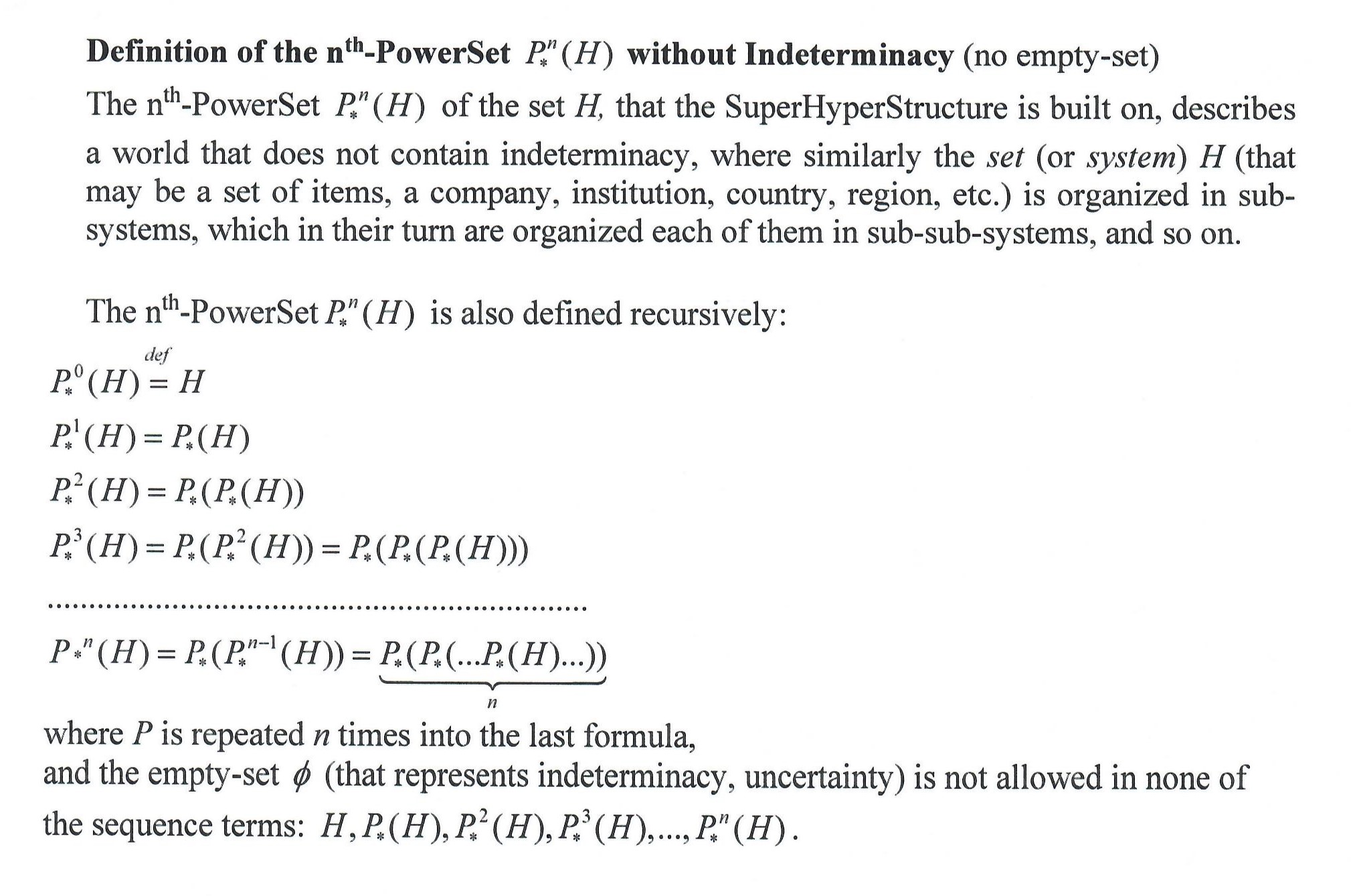
    The n-th PowerSet Pⁿ(H) and P_*ⁿ(H) of a non-empty set H, were introduced by Smarandache [2] in 2016.

    5. Particular cases of SuperHyperStructure [15] and respectively Neutrosophic SuperHyperStructure

Depending on the type of Structure, in any field of knowledge, on has multiple types of SuperHyperStructures and respectively their corresponding Neutrosophic SuperHyperStructures.  Such as:

- SuperHyperAlgebra (when the structure is an Algebra), SuperHyperGraph (when the structure is a Graph), SuperHyperGeometry (when the structure is a type of Geometry), SuperHyperCalculus etc.

Further on, a SuperHyperAlgebra may be as particular cases: SuperHyper Semigroup, SuperHyper Group, SuperHyper Field etc.

A SuperHyperGeometry may be as particular cases: SuperHyper EuclideanGeometry, Superhyper NonEuclideanGeometry, SuperHyper ProjectiveGeometry, etc.

   References

[1] F. Marty, Sur une généralisation de la Notion de Groupe, 8th Congress Math. Scandinaves, Stockholm,        Sweden, (1934), 45–49.

[2] F. Smarandache, SuperHyperAlgebra and Neutrosophic SuperHyperAlgebra, Section into the authors book Nidus Idearum. Scilogs, II: de rerum consectatione, Second Edition, (2016), pp. 107– 108, https://fs.unm.edu/NidusIdearum2-ed2.pdf

[3] Florentin Smarandache, HyperUncertain, SuperUncertain, and SuperHyperUncertain  Sets/Logics/Probabilities/Statistics, Critical Review, Vol. XIV, 2017, 10-19.

     [4] Abdullah Kargın, Florentin Smarandache, Memet Şahin: New Type Hyper Groups, New Type SuperHyper

Groups and Neutro-Type SuperHyper Groups. Chapter One in Florentin Smarandache, Memet Şahin, Derya

Bakbak, Vakkas Uluçay & Abdullah Kargın (Editors) - Neutrosophic SuperHyperAlgebra And New Types of

Topologies, Global Knowledge, pp. 10-24, 2023.

[5] Florentin Smarandache, Extension of HyperGraph to n-SuperHyperGraph and to Plithogenic n-SuperHyperGraph, and Extension of HyperAlgebra to n-ary (Classical-/Neutro-/Anti-) HyperAlgebra, Neutrosophic Sets and Systems, vol. 33, 2020, pp. 290-296. DOI: 10.5281/zenodo.3783103

[6] S Santhakumar, I R Sumathi and J Mahalakshmi J, A Novel Approach to the Algebraic Structure of Neutrosophic SuperHyper Algebra , Neutrosophic Sets and Systems, Vol. 60, 2023, pp. 593-602. DOI: 10.5281/zenodo.10236475

[7] Sirus Jahanpanah and Roohallah Daneshpayeh, On Derived SuperHyper BE-Algebras, Neutrosophic Sets and Systems, Vol. 57, 2023, pp. 318-327. DOI: 10.5281/zenodo.8271390

[8] Marzieh Rahmati and Mohammad Hamidi, Extension of G-Algebras to SuperHyper G-Algebras, Neutrosophic Sets and Systems, Vol. 55, 2023, pp. 557-567. DOI: 10.5281/zenodo.7879543

[9] Mohammad Hamidi, On Superhyper BCK-Algebras, Neutrosophic Sets and Systems, Vol. 53, 2023, pp. 580-588. DOI: 10.5281/zenodo.7536091

[10] Huda E. Khali , Gonca D. GÜNGÖR, Muslim A. Noah Zainal^,  Neutrosophic SuperHyper Bi-Topological Spaces: Original Notions and New Insights, Neutrosophic Sets and Systems, Vol. 51, 2022, pp. 33-45. DOI: 10.5281/zenodo.7135241

[11] Pairote Yiarayong, On 2-SuperHyperLeftAlmostSemihyp regroups, Neutrosophic Sets and Systems, Vol. 51, 2022, pp. 516-524. DOI: 10.5281/zenodo.713536

[12] Florentin Smarandache, The SuperHyperFunction and the Neutrosophic  SuperHyperFunction, Neutrosophic Sets and Systems, Vol. 49, 2022, pp. 594-600. DOI: 10.5281/zenodo.6466524

[13] F. Smarandache, Introduction to the n-SuperHyperGraph - the most general form of graph today, Neutrosophic Sets and Systems, Vol. 48, 2022, pp. 483-485, DOI: 10.5281/zenodo.6096894

     [14] Florentin Smarandache, Foundation of Revolutionary Topologies: An Overview, Examples, Trend

     Analysis, Research Issues, Challenges, and Future Directions [including SuperHyperTopology], Neutrosophic