Innovative Efficiency Measurement Using Stackelberg Games and Neutrosophic Numbers

Abstract

This paper addresses the challenge of measuring efficiency in systems with two 
components using Stackelberg game theory and neutrosophic numbers to handle data uncertainty. 
Unlike traditional Data Envelopment Analysis (DEA) models that treat Decision Making Units 
(DMUs) as black boxes, we propose a new neutrosophic Network DEA (NDEA) model to compute 
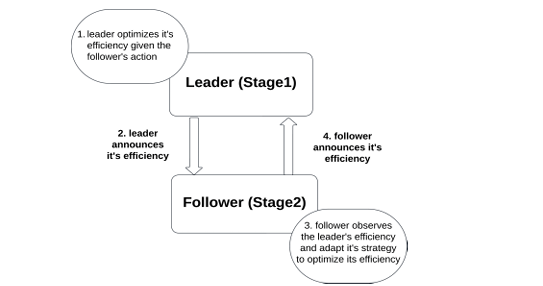
efficiency scores for each division. By applying Stackelberg game theory, we break down the 
system's efficiency scores into sub-system efficiencies, providing a more detailed assessment of 
performance. Our proposed model represents a significant contribution to the literature by 
developing a two-stage Network Slack Based Measure (NSBM) model. This model addresses 
conflicts arising from the dual role of intermediate measures and assumes a Stackelberg-game 
relationship between the two stages while ensuring continuity in the flow of links between them. 
Additionally, the model imposes a penalty on the follower's objective to discourage deviation from 
the leader's objectives, thereby enhancing feasibility robustness. To handle uncertainty within the 
model, we further refine it into a two-stage framework that leverages Pareto efficiency concepts to 
establish lower and upper bounds for DMU efficiencies. To validate our approach, we utilized data 
from the Iranian Airline Industry. This empirical study evaluates the efficiencies of 13 Iranian 
airlines, illustrating how our methodology effectively captures and quantifies operational 
efficiencies within uncertainty. 

DOI: 10.5281/zenodo.14708763