Approach to Multi-Criteria Decision-Making in a Neutrosophic  Picture Hyper-Soft Set Environment using Generalized  Neutrosophic TOPSIS

Manpreet Kaur; Akanksha Singh

Authors

Manpreet Kaur Department of Mathematics, Chandigarh University, Gharuan, Mohali, India;
Akanksha Singh Department of Mathematics, Chandigarh University, Gharuan, Mohali, India;

Keywords:

Picture fuzzy set, soft set, Hyper-soft set, Neutrosophic set

Abstract

Given the complexity of today’s world, we might need to work with numbers requiring
multi-attribute functions, such as those having positive, neutral, and negative membership and
those having truth, indeterminacy, and falsity membership. Adding these numbers together to get
a single real number is the most important factor. In situations like this, decision-makers have
more difficult choices and are unable to apply the single attribute function of the soft set
theory. To address this constraint, the hyper-soft set theory with multi-attribute functions is
introduced. We combine the notion of neutrosophic hypersoft set with picture fuzzy hypersoft set
to form a single theory of neutrosophic picture hypersoft set in this study. We introduce the
notions of correlation coefficient and weighted correlation coefficient and demonstrate its
fundamental properties for neutrosophic picture hypersoft set. Then, we introduce the notions of a
weighted average operator and a weighted geometric operator for neutrosophic picture hypersoft
set by using the various aggregation operators with a suitable example. Making decisions based
on several factors and choosing the best option is multi-criteria decision-making or MCDM. When
ranking and choosing options based on a distance metric, one essential and useful strategy is the
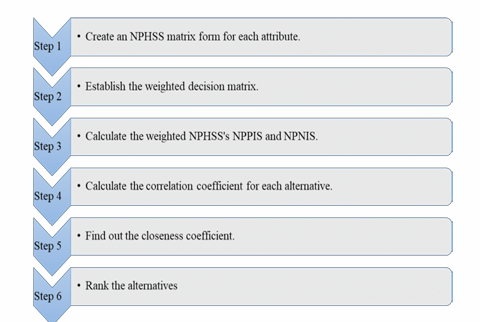
Technique of order preference by similarity to an ideal solution (TOPSIS). We demonstrate the
accuracy of the fuzzy TOPSIS methodology by extending it to neutrosophic fuzzy TOPSIS and
using neutrosophic picture hypersoft set theory to describe the MCDM problem in this study. We
provide a generalized neutrosophic TOPSIS approach to demonstrate correlation coefficients and
the effectiveness of this approach with an appropriate example. Finally, we offer a comparison to
prior studies to demonstrate the viability of the proposed approach.