Modified Non-Linear Triangular Neutrosophic Numbers: Theory and Applications in Integral Equation

Abstract

Existing methods for handling uncertainty and imprecision often fall short in addressing complex
real-world problems. To overcome these limitations, this paper introduces a novel Generalized Non-Linear
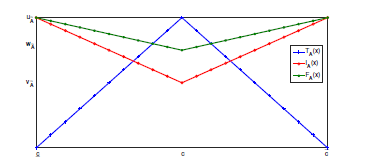
Triangular Neutrosophic Number (GNLTNN) that effectively captures uncertainty, indeterminacy, and falsity.
By analysing GNLTNN through (α,β,γ)-cuts and defining arithmetic operations using the max-min principle,
we provide a robust framework for handling neutrosophic information. The proposed neutrosophic Laplace
transform method enables efficient solutions to integral equations involving non-linear neutrosophic numbers.
The efficacy of our approach is demonstrated through graphical representations

DOI: 10.5281/zenodo.13566880