Inventory Control of Single Perishable Item in Neutrosophic Environment: with Stock-based Demand, Preservation technology, Shortage and Two-stage price discount for Imperfect items

Abstract

 In this article, we study an inventory model for a single perishable item which can contribute to
 economic growth, organizational benefit as well as individual life. Both supplier and retailer consider price
 discount for defective items up to a specified percentage, in a situation where the parameters involved are
 vague and imprecise. Such consideration has not been reported in the literature. By recognizing the power of
 neutrosophic environment to handle uncertainty and impreciseness inherited or existing in the problem, we use
 single-valued triangular neutrosophic numbers (SVNs) for representing stock-based demand, retail price, order
ing cost, holding cost, wastage cost, imperfect item price, and preservation cost with de-neutrosophication. The
 Taylor series approximation is used to transform the time variables in the profit function into the order quantity
 and maximum stock variables. We determine the theoretical optimality condition and verify the concavity and
 uniqueness of the global optimal solution using Hessian matrix. Our aim is to determine the maximum value of
 the retailer’s profit by making decisions on the order quantity and maximum stock level, as well as to estimate
 shortages, stock-out time, cycle length, and identify the model’s inherent impreciseness. We develop neutro
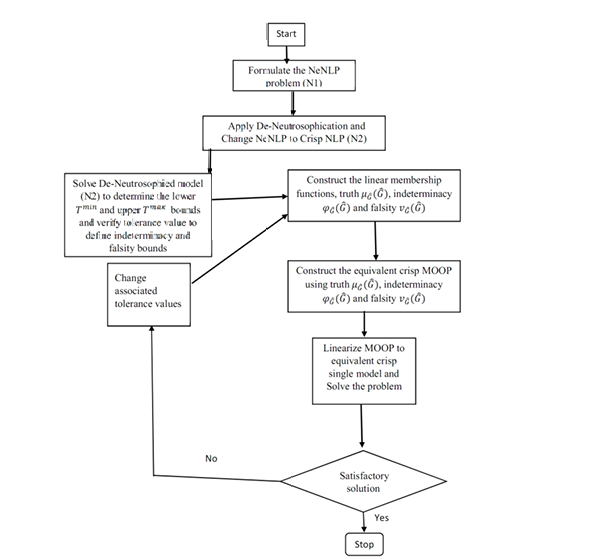
sophic fuzzy optimization technique ( NeFOT), an extension of the intuitionistic fuzzy optimization technique
 (IFOT) to evaluate the compromise acceptance, indeterminacy, and rejection levels inherited in the model and
 optimal solution. We support the study by illustrating a numerical example and graph with Mathematica 11.3
 and LINGO 18.0.

DOI: 10.5281/zenodo.14120586