Solving the shortest path based on the traveling salesman problem with a genetic algorithm in a Fermatean neutrosophic environment
Keywords:
Fermatean neutrosophic number; Genetic algorithm; Traveling salesman problem; Shortest path problemAbstract
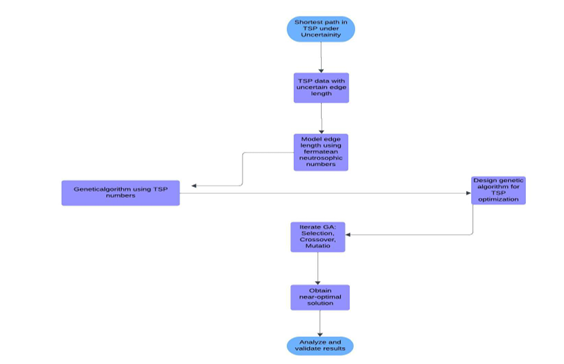
The Traveling Salesman Problem (TSP) is one of the most significant and well-known
optimization problem that is frequently limited by uncertainty in edge lengths. Existing methods
fail to effectively model and solve such problems in uncertain environments. To address this gap,
we propose a novel approach that combines a genetic algorithm (GA) with Fermatean neutrosophic
numbers to provide a more robust representation of uncertainty. This work presents a
comprehensive framework for evaluating the shortest path in a given network by precisely
characterizing uncertain edge lengths. The proposed methodology is tested on various TSP
scenarios of varying complexities, demonstrating its ability to generate near-optimal solutions with
higher efficiency and accuracy than traditional techniques. Our findings highlight the method's
potential for advancing uncertain route optimization and have significant practical implications for
real-world logistics.
Downloads
Downloads
Published
Issue
Section
License
Copyright (c) 2024 Neutrosophic Sets and Systems

This work is licensed under a Creative Commons Attribution 4.0 International License.







