A Study on Continuity functions in Neutro-Topological Spaces
Keywords:
Neutro-topological space, neutro-continuity, weakly neutro-continuity, neutro-open map, neutro-closed map, neutro-homeomorphismAbstract
The specific purpose of this study is to define continuity of mappings in
neutro-topological spaces using neutro-open and neutro-closed sets and analyze the properties of
continuous functions that are true in classical topological spaces in the neutro-topological space.
Neutro-interior and neutro-closure in neutro-topological spaces have some properties that are
somewhat different from those in classical topological spaces. However, with the definition of a
new form of continuity, termed as weakly neutro-continuity, much of the properties of continuous
functions could be established in neutro-topological spaces. Neutro-open map and neutro-closed
maps are also defined on the basis of neutro-open and neutro-closed sets. The notion of weakly
neutro-continuity has been used to define neutro-homeomorphism and many of the properties of
homeomorphism are analyzed and found to be true in the case of neutro-homeomorphism. A
comparison of some of the properties of continuity and homeomorphism in classical topological
spaces have been done vis-à-vis the neutrosophic topological spaces and neutro-topological spaces.
Downloads
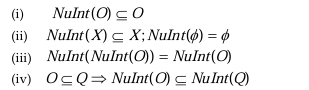
Downloads
Published
Issue
Section
License
Copyright (c) 2024 Neutrosophic Sets and Systems

This work is licensed under a Creative Commons Attribution 4.0 International License.






